Assume again that g = Pf is known for 
.
We want to derive an inversion procedure similar to the one in section 2.1. With the backprojection
we have again
provided that 
. Again the convolutions on the left hand right hand side have
different meanings. Explicitly this reads
which corresponds to (2.2). As in (2.2) we express the relationship
in Fourier space, obtaining
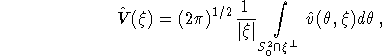
see Colsher (1980). In order to get an inversion formula for P we have to determine v such that 
or 
, i.e.
A solution 
independent of 
is
where 
is the length of 
. For the spherical zone 
from section 3.4 with 
, 
, 
a constant with 
, Colsher computed 
explicitly. With 
be obtained
Filters such as the Colsher filter (3.10) do not have small support. This means that g in (3.8) has to be known in all of 
. Often g is only available
in part of (truncated projections). Let us choose
where 
from section (3.4) and 
is a horizontal unit vector. Since (3.11) is constant in the vertical direction, v is a 
-function in the vertical variable. Hence the
integral on the right hand side of (3.8) reduces to an integral over horizontal lines in , making it possible to handle truncated projections. Unfortunately,
(3.11) does not quite satisfy , i.e. it does not provide an exact inversion. Instead we only have
where 
, 
.
This is close to if is small. In this case reconstruction from truncated projections is possible, at least approximately.
