Accelerated beams & lattices
In order to tune the effects of light-matter interaction, we study the artificial creation of refractive index structures and the subsequent light propagation in these photonic lattices. Therefore, huge efforts have been made to tailor light fields in any desired manner, since their spatial intensity distributions are capable to inscribe corresponding refractive index modulations in photosensitive materials.
In the recent years, the focus shifts from periodic intensity modulations, over random structures, to the fascinating topic of accelerated beams. The most prominent representative of this class of accelerated beams is the Airy beam, which is imaged beneath. Its transverse intensity distribution stays constant during propagation, while the complete pattern moves on a parabolic trajectory due to a constant acceleration.
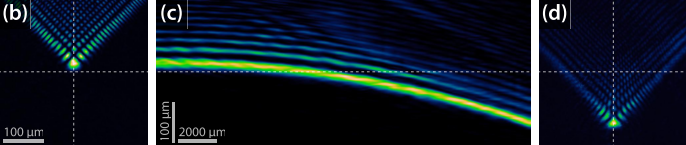
We focused on several groundbreaking realizations that became possible due to this unique propagation effect:
- Optical information processing requires light control: Optical storage, interconnections, and optical routers are highly desired photonic components. Therefore, we investigated light routing in Airy beam induced waveguides, capable to flexibly guide an incoming signal to different outputs.
- Full control over the trajectory of the Airy beam requires additionally, that we gain control over the acceleration of the transverse intensity distribution. Thus, we optically induced a periodic diamond lattice in photorefractive SBN and demonstrated the hindrance of the curvature of the parabolic trajectory, in dependence of the lattice strength.
- Highly appealing is the nonlinear interaction of two Airy beams that are accelerated towards a common focal point. At the merging point, their coherent constructive interference leads to high intensities that nonlinearly compensate for the typical diffraction every light field is subjected to, and form a straightly propagating nondiffracting soliton.
These realizations and the Airy beam’s promising properties are reasons for us to investigate further nonlinear effects.

