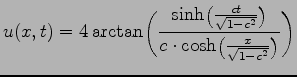The sine-Gordon equation is a nonlinear hyperbolic partial differential equation involving the d'Alembert operator and the sine of the unknown function. It was originally considered in the nineteenth century in the course of study of surfaces of constant negative curvature. The equation grew greatly in importance when it was realized that it led to solitons ( so-called ''kink`` and ''antikink``). The equation reads
 |
(2.18) |
where 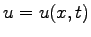 .
An interesting feature of the sine-Gordon equation is the existence of soliton and multisoliton solutions. If we look for localized waves of permanent profile of the form
.
An interesting feature of the sine-Gordon equation is the existence of soliton and multisoliton solutions. If we look for localized waves of permanent profile of the form
 , such as
, such as
 and
and
 , when
, when
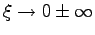 , the one-soliton solution can be calculated
, the one-soliton solution can be calculated
 |
(2.19) |
Equation (2.19) represents a localized solitary wave, travelling at any velocity
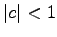 . The
. The 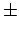 signs correspond to localized solutions which are called kink and antikink correspondenly (see Fig. 2.2).
signs correspond to localized solutions which are called kink and antikink correspondenly (see Fig. 2.2).
Figure 2.8:
Representation of the kink (blue) and antikink (red) solutions (2.19)
 |
The kink-kink collision solution has the form
 |
(2.20) |
and describes the collision between two kinks with respective velocities 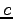 and
and 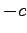 and approaching the origin from
and approaching the origin from
 and moving away from it with velocities
and moving away from it with velocities 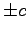 for
for
 .
.
Figure 2.9:
The kink-kink collision, calculated at three different times: At 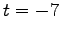 (red curve) both kinks propagate with opposite velocities
(red curve) both kinks propagate with opposite velocities 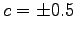 ; At
; At 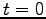 they collide at the origin (green curve); At
they collide at the origin (green curve); At 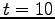 (blue curve) they move away from the origin with velocities
(blue curve) they move away from the origin with velocities 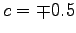 .
.
 |
Moreover, one can construct solution, corresponding to the kink-antikink coliision. The solution reads:
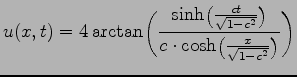 |
(2.21) |
The breather soliton solution, which is also called a breather mode or breather soliton, is given by
 |
(2.22) |
which is periodic for frequencies 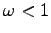 and decays exponentially when moving away from
and decays exponentially when moving away from  .
.
Figure 2.10:
The breather solution, oscillating with the frequency
 , calculated for three different times
, calculated for three different times 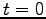 (red curve),
(red curve), 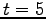 (green curve) and
(green curve) and 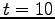 (blue curve).
(blue curve).
 |
Subsections
Gurevich_Svetlana
2008-11-12